A Man, a Mystery, and a Million Dollars: A Brief History of the Riemann Hypothesis (part 2)

(part one can be found here; it is highly recommended that you read it before coming here)

Now: a history of this hypothesis.

Introducing: Bernhard Riemann!

Riemann is the guy behind the Riemann hypothesis. He did some other things in math too apart from that (the phrase Riemann integral may leap to mind for calculus students, and there is a whole Wikipedia article titled “List of things named after Bernhard Riemann”), but for now I’ll focus on his connection with the hypothesis.

So some time ago, in ’59 (1859, that is), Riemann wrote a paper. This paper was titled Ueber die Anzahl der Primzahlen unter einer gegebenen Grösse¹Yes, that’s Ueber, not Über. I’m not a linguist myself, but from what I can tell, this is simply an archaic variant that means the same thing..

…

…sorry. For those of you who don’t speak German, that’s On the Number of Primes Less Than a Given Magnitude in English. Riemann starts the paper (after one paragraph) thus:

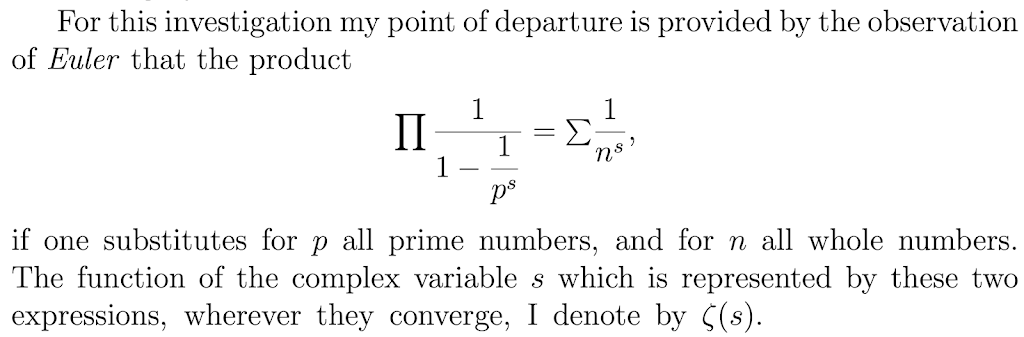
Riemann actually invented the notation \zeta(s) for the Riemann zeta function, which is why it bears his name (as opposed to that of Euler). It then goes on to explore number theory and the titular topic, but we are interested in this part:

(When he was talking about the roots being “real”, he was talking about a modified version of the zeta function, the Riemann xi function²The Riemann xi function, \xi(s), is given by \xi(s)=\Pi\left(\frac{t}{2}\right)(t-1)\pi^{-\frac{t}{2}}\zeta(t) where t=\frac{1}{2}+si and \Pi(x) is what Desmos gives you when you type “x!” into the box. The Riemann hypothesis implies the roots of the xi function are real.)

Riemann didn’t, of course, know that his “fleeting futile attempts” would culminate in a rabid search to prove it, which would become a literal million-dollar question.

As of this publishing, it remains unsolved. If you’re willing to give it a shot, give it a shot! You can do anything if you put your mind to it!³Except for this. You cannot do this. I mean, probably thousands of people have tried and failed before you, but still!

Sources/Footnotes

• 1
  Yes, that’s Ueber, not Über. I’m not a linguist myself, but from what I can tell, this is simply an archaic variant that means the same thing.
• 2
  The Riemann xi function, \xi(s), is given by \xi(s)=\Pi\left(\frac{t}{2}\right)(t-1)\pi^{-\frac{t}{2}}\zeta(t) where t=\frac{1}{2}+si and \Pi(x) is what Desmos gives you when you type “x!” into the box.
• 3
  Except for this. You cannot do this.